Ventilation & Masks: Reducing Airborne Transmission of COVID-19 in a Classroom

Author Eric A. Schiff

Download the Full Article

Key Findings

  • In a classroom setting with low ventilation and unmasked students, a superspreader’s COVID-19 infection will spread to essentially the entire class.
  • Neither good ventilation nor good masking, acting alone, reduces the percentage of students infected below 10%
  • Used in conjunction, good ventilation and masking reduced the calculated infection percentage to 2%.
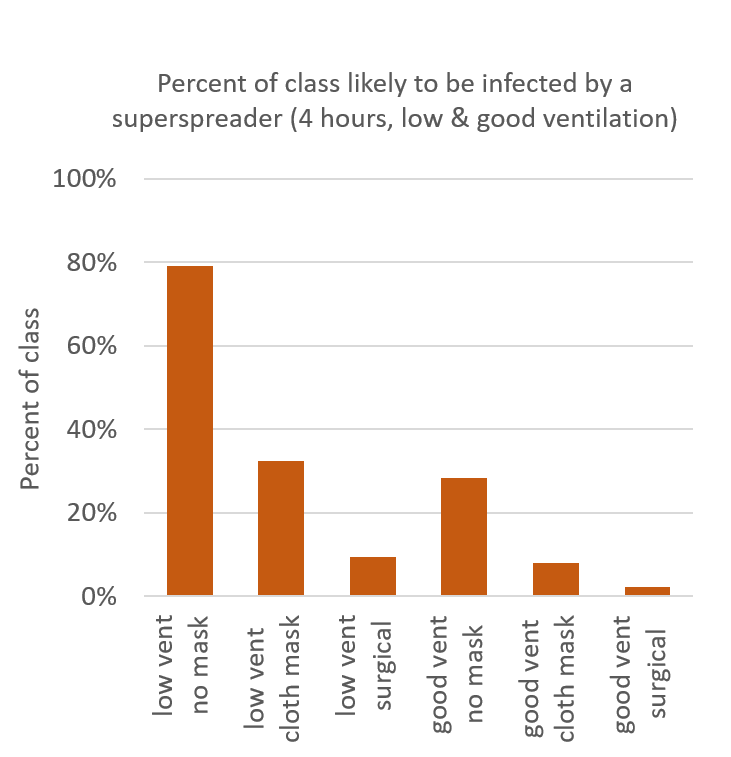
Bar chart showing calculations for the likely percentage of a class that will be infected after 4 hours with a COVID-19 superspreader. Three levels of masking are shown. Low ventilation is one clean air change per hour, which applies in some schools. Good ventilation is 6 air changes per hour.

Summary

We’ve calculated the number of COVID-19 infections that will be spread from a single COVID-19 “superspreader” to students and teachers in a classroom shared for 4 hours. Without masking and with a low ventilation rate, nearly all susceptible students and teachers will be infected. Neither masking nor ventilation alone is sufficient to reduce the infection rate below 10%. Careful use of surgical masks along with good ventilation reduced the estimated infection rate to 2%. The bar chart below presents the estimated infection rates for low and for good ventilation, and for unmasked, cloth masked, and surgically masked students and teachers. The estimates are based on a comparison with the Guangzhou restaurant cluster of COVID-19 infections, and use the “Wells-Riley” model to calculate infection rates.

Acknowledgments

The author thanks Jensen Zhang (Syracuse University) for a critical reading of the manuscript. The information, data, or work presented herein was funded in part by an award from the New York State Department of Economic Development (DED) to the Center of Excellence in Environmental & Energy Systems (SyracuseCoE) at Syracuse University. Any opinions, findings, conclusions or recommendations expressed are those of the author(s) and do not necessarily reflect the views of the DED.

About the Author

Eric A. Schiff, Ph.D., is interim director of SyracuseCoE (Syracuse Center of Excellence in Environmental and Energy Systems) and Professor in the Department of Physics at Syracuse University. email: easchiff@syr.edu .

References

1 Yuguo Li, et al., “Evidence for probable aerosol transmission of SARS-CoV-2 in a poorly ventilated restaurant”, medRxiv preprint https://doi.org/10.1101/2020.04.16.20067728.

2 Jensen Zhang, “Integrating IAQ control strategies to reduce the risk of asymptomatic SARS CoV-2 infections in classrooms and open plan offices”, Science and Technology for the Built Environment 26, 1013-1018 (2020), DOI: 10.1080/23744731.2020.1794499. The effective ventilation rate Q is the product of the actual air flow and a factor that allows for imperfect removal or denaturing of virions in recirculated air.

3 J. Ma, et al., “Exhaled breath is a significant source of SARS-CoV-2 emission”, medRxiv preprint https://doi.org/10.1101/2020.05.31.20115154.

4 The air changes per hour (ach) is calculated as 60 x Q/V, where the units of Q are cubic feet per minute and V is the room’s volume (in cubic feet).

5 The original Wells-Riley form assumes a time-independent density of airborne virus; we have generalized the calculation to include the initial increase of the virion density following the arrival of the superspreader in the room. The use of a relative exposure presumes that classroom occupants and the diners in Guangzhou have similar inhalation rates and other factors of disease susceptibility. The Wells-Riley concept of a “quantum” for airborne transmission of disease is commonly assumed in studies of ventilation effects on infections, but other forms have been studied. See G. N. Sze To, C. Y. H. Chao, “Review and comparison between the Wells–Riley and dose-response approaches to risk assessment of infectious respiratory diseases”, Indoor Air 20, 2–16 (2010). https://www.ncbi.nlm.nih.gov/pmc/articles/PMC7202094/#b51 ; doi: 10.1111/j.1600-0668.2009.00621.x

6 W. R. Chan, et al., “Ventilation rates in California classrooms: Why many recent HVAC retrofits are not delivering sufficient ventilation”, Building and Environment 167, 106426 (2020). https://doi.org/10.1016/j.buildenv.2019.106426

7 A. Mueller and L. Fernandez, “Assessment of Fabric Masks as Alternatives to Standard Surgical Masks in Terms of Particle Filtration Efficiency”, medRxiv preprint doi: https://doi.org/10.1101/2020.04.17.20069567.

8 M. Klompas, M. A. Baker, C. Rhee, “Airborne Transmission of SARS-CoV-2: Theoretical Considerations and Available Evidence”, JAMA 324(5): 441-442. doi:10.1001/jama.2020.12458.

Print 🖨 PDF 📄